Mathematics Level 2 Test Form B Answers
SAT Math 1 & 2 Subject Tests
Chapter 16
Mathematics Level 2 Practice Test Form B
Click here to download a PDF of the Mathematics Level 2 Practice Test Form B.
![]()
MATHEMATICS LEVEL 2 TEST FORM B
For each of the following problems, decide which is the BEST of the choices given. If the exact numerical value is not one of the choices, select the choice that best approximates this value. Then fill in the corresponding oval on the answer sheet.
Notes: (1) A scientific or graphing calculator will be necessary for answering some (but not all) of the questions on this test. For each question, you will have to decide whether or not you should use a calculator.
(2) For some questions in this test you may have to decide whether your calculator should be in the radian mode or the degree mode.
(3) Figures that accompany problems on this test are intended to provide information useful in solving the problems. They are drawn as accurately as possible EXCEPT when it is stated in a specific problem that its figure is not drawn to scale. All figures lie in a plane unless otherwise indicated.
(4) Unless otherwise specified, the domain of any function f is assumed to be the set of all real numbers x for which f(x) is a real number. The range of f is assumed to be the set of all real numbers f(x), where x is in the domain of f.
(5) Reference information that may be useful in answering the questions on this test can be found below.
THE FOLLOWING INFORMATION IS FOR YOUR REFERENCE IN ANSWERING SOME OF THE QUESTIONS ON THIS TEST.
Volume of a right circular cone with radius r and height h: V = ![]() πr 2 h
πr 2 h
Lateral area of a right circular cone with circumference of the base c and slant height ℓ: S = ![]() cl
cl
Volume of a sphere with radius r: V = ![]() πr 3
πr 3
Surface area of a sphere with radius r: S = 4πr 2
Volume of a pyramid with base area B and height h: V = ![]() Bh
Bh
1. If xy ≠ 0 and 3x = 0.3y, then ![]() =
=
(A) 0.1
(B) 1.0
(C) 3.0
(D) 9.0
(E) 10.0
2. If f(x) = (3![]() − 4)2, then how much does f(x) increase as x goes from 2 to 3 ?
− 4)2, then how much does f(x) increase as x goes from 2 to 3 ?
(A) 1.43
(B) 1.37
(C) 1.00
(D) 0.74
(E) 0.06
3. What is the equation of a line with a y-intercept of 3 and an x-intercept of −5 ?
(A) y = 0.6x + 3
(B) y = 1.7x − 3
(C) y = 3x + 5
(D) y = 3x − 5
(E) y = −5x + 3
4. For what positive value of a does a − ![]() equal −4 ?
equal −4 ?
(A) 0.56
(B) 1.00
(C) 1.12
(D) 2.06
(E) 4.12
5. If the second term in an arithmetic sequence is 4, and the tenth term is 15, what is the first term in the sequence?
(A) 1.18
(B) 1.27
(C) 1.38
(D) 2.63
(E) 2.75
6. If g(x) = |5x 2 − x 3|, then g(6) =
(A) −54
(B) −36
(C) 36
(D) 216
(E) 396
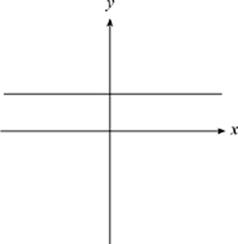
7. Which of the following graphs of functions is symmetrical with respect to the line y = x ?
(A)
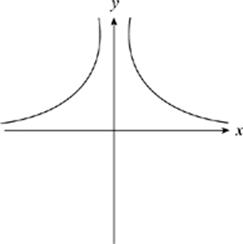
(B)
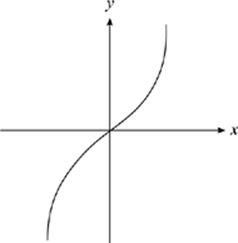
(C)
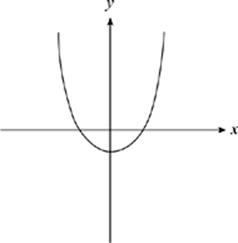
(D)
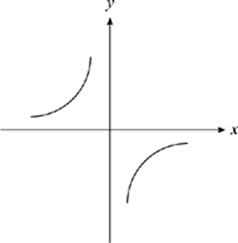
(E)
8. If 0° ≤ A ≤ 90° and sin A =![]() sin 75°, then A =
sin 75°, then A =
(A) 12.9º
(B) 18.8º
(C) 25.0º
(D) 32.2º
(E) 45.0º
9. If f(x) = ![]() x 2 − 6x + 11, then what is the minimum value of f(x) ?
x 2 − 6x + 11, then what is the minimum value of f(x) ?
(A) −8.0
(B) −7.0
(C) 3.2
(D) 6.0
(E) 11.0
10. |x − y| + |y − x|
(A) 0
(B) x − y
(C) y − x
(D) 2|x − y|
(E) 2|x + y|
0° ≤ A ≤ 90°
0° ≤ B ≤ 90°
11. If sin A = cos B, then which of the following must be true?
(A) A = B
(B) A = 2B
(C) A = B + 45
(D) A = 90 − B
(E) A = B + 180
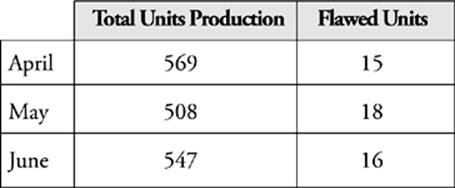
Figure 1
12. Each month, some of the automobiles produced at the Carco plant have flawed catalytic converters. According to the chart in Figure 1, what is the probability that a car produced in one of the three months shown will be flawed?
(A) 0.01
(B) 0.02
(C) 0.03
(D) 0.04
(E) 0.05

Figure 2
13. Adamsvillle building codes require that a wheelchair ramp must rise at an angle (θ) of no less than 5° and no more than 7° from the horizontal. If a wheelchair ramp rises exactly 3 feet as shown in Figure 2, which of the following could be the length of the ramp?
(A) 19.0 feet
(B) 24.0 feet
(C) 28.0 feet
(D) 35.0 feet
(E) 42.0 feet
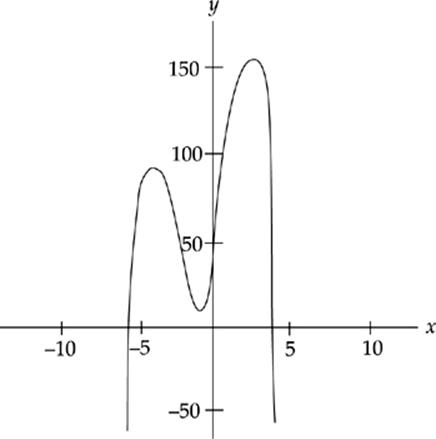
Figure 3
14. Figure 3 represents the graph of the function y = −x 4 − 4x 3 + 14x 2 + 45x − n. Which of the following could be the value of n ?
(A) −50
(B) −18
(C) 50
(D) 100
(E) 150
15. What value does 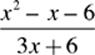 approach as x approaches −2 ?
approach as x approaches −2 ?
(A) −1.67
(B) −0.60
(C) 0
(D) 1.00
(E) 2.33
16. In Titheland, the first 1,000 florins of any inheritance are untaxed. After the first 1,000 florins, inheritances are taxed at a rate of 65%. How large must an inheritance be, to the nearest florin, in order to amount to 2,500 florins after the inheritance tax?
(A) 7,143
(B) 5,286
(C) 4,475
(D) 3,475
(E) 3,308
17. In an engineering test, a rocket sled is propelled into a target. If the sled"s distance d in meters from the target is given by the formula d = −1.5t 2 + 120, where t is the number of seconds after rocket ignition, then how many seconds have passed since rocket ignition when the sled is 10 meters from the target?
(A) 2.58
(B) 8.56
(C) 8.94
(D) 9.31
(E) 11.26
18. ![]() 3k − 2 =
3k − 2 =
(A) 25
(B) 28
(C) 145
(D) 280
(E) 290
19. If ex = 5, then x =
(A) 0.23
(B) 1.61
(C) 7.76
(D) 148.41
(E) 13.59
20. If the greatest possible distance between two points within a certain rectangular solid is 12, then which of the following could be the dimensions of this solid?
(A) 3 × 3 × 9
(B) 3 × 6 × 7
(C) 3 × 8 × 12
(D) 4 × 7 × 9
(E) 4 × 8 × 8
21. Runner A travels a feet every minute. Runner B travels b feet every second. In one hour, runner A travels how much farther than runner B, in feet?
(A) a − 60b
(B) a 2 − 60b 2
(C) 360a − b
(D) 60(a − b)
(E) 60(a − 60b)
22. A right triangle has sides in the ratio of 5 : 12 : 13. What is the measure of the smallest angle in the triangle, in degrees?
(A) 13.34
(B) 22.62
(C) 34.14
(D) 42.71
(E) 67.38
23. If f(x) - ![]() , and g(x) =
, and g(x) = ![]() + 1, then g(f(x)) =
+ 1, then g(f(x)) =
(A) 2
(B) x + 2
(C) 2x + 2
(D) ![]()
(E) ![]()
24. If f(x) = (x − π)(x − 3)(x − e), then what is the greatest possible distance between points at which the graph of y = f(x) intersects the x-axis?
(A) 0.14
(B) 0.28
(C) 0.36
(D) 0.42
(E) 0.72
25. 
(A) 0.5
(B) 2.0
(C) x
(D) x 2 − x
(E) x 2 − 2x + 1
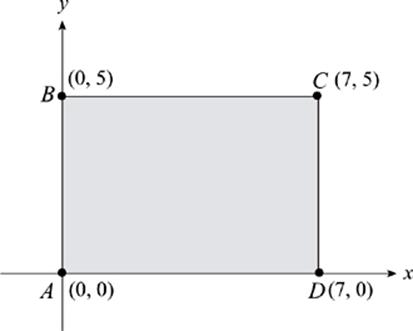
Figure 4
26. What is the volume of the solid created by rotating rectangle ABCD in Figure 4 around the y-axis?
(A) 219.91
(B) 245.00
(C) 549.78
(D) 769.69
(E) 816.24
27. If f(x, y) = 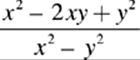 , then f(−x, −y) =
, then f(−x, −y) =
(A) 1
(B) ![]()
(C) 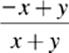
(D) 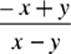
(E) 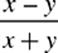
28. In order to disprove the hypothesis, "No number divisible by 5 is less than 5," it would be necessary to
(A) prove the statement false for all numbers divisible by 5
(B) demonstrate that numbers greater than 5 are often divisible by 5
(C) indicate that infinitely many numbers greater than 5 are divisible by 5
(D) supply one case in which a number divisible by 5 is less than 5
(E) show that a statement true of numbers greater than 5 is also true of numbers less than 5
29. A parallelogram has vertices at (0, 0), (5, 0), and (2, 3). What are the coordinates of the fourth vertex?
(A) (3, −2)
(B) (5, 3)
(C) (7, 3)
(D) (10, 5)
(E) It cannot be determined from the information given.
30. The expression 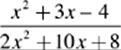 is undefined for what values of x ?
is undefined for what values of x ?
(A) x = {−1, −4}
(B) x = {−1}
(C) x = {0}
(D) x = {1, −4}
(E) x = {0, 1, 4}
31. For which of the following functions is f(x) > 0 for all real values of x ?
I. f(x) = x 2 + 1
II. f(x) = 1 − sin x
III. f(x) = π (π x − 1)
(A) I only
(B) II only
(C) I and III only
(D) II and III only
(E) I, II, and III

Figure 5
32. The graph of y = f(x) is shown in Figure 5. Which of the following could be the graph of y = −f(−x) ?
(A)

(B)
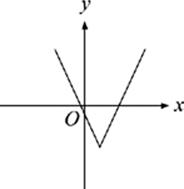
(C)
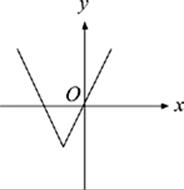
(D)
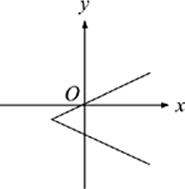
(E)
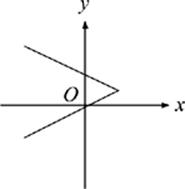
33. A wire is stretched from the top of a two-foot-tall anchor to the top of a 50-foot-tall antenna. If the wire is straight and has a slope of ![]() , then what is the length of the wire in feet?
, then what is the length of the wire in feet?
(A) 89.18
(B) 120.00
(C) 123.26
(D) 129.24
(E) 134.63
34. If ![]() > θ 2π and sec θ = 4, then tan θ =
> θ 2π and sec θ = 4, then tan θ =
(A) −3.93
(B) −3.87
(C) 0.26
(D) 3.87
(E) 3.93
35. Circle O is centered at (−3, 1) and has a radius of 4. Circle P is centered at (4, −4) and has a radius of n. If circle O is externally tangent to circle P, then what is the value of n ?
(A) 4.00
(B) 4.37
(C) 4.60
(D) 5.28
(E) 6.25
36. In triangle ABC, ![]() =
=![]() and
and ![]() =
= ![]() . If angles A, B, and C are opposite sides a, b, and c, respectively, and the triangle has a perimeter of 16, then what is the length of a ?
. If angles A, B, and C are opposite sides a, b, and c, respectively, and the triangle has a perimeter of 16, then what is the length of a ?
(A) 2.7
(B) 4.7
(C) 5.3
(D) 8.0
(E) 14.0
37. The table of values above shows selected coordinate pairs on the graph of h(x). Which of the following could be h(x) ?
(A) x(x + 1)(x − 1)
(B) (x + 1)2(x − 1)
(C) (x − 1)(x + 2)2
(D) (x − 1)2(x + 3)
(E) (x − 1)(x + 1)(2x − 3)
a + b + 2c = 7
a − 2b = 8
3b + 2c = n
38. For what values of n does the system of equations above have no real solutions?
(A) n ≠ −1
(B) n ≤ 0
(C) n ≥ 1
(D) n > 7
(E) n = −15
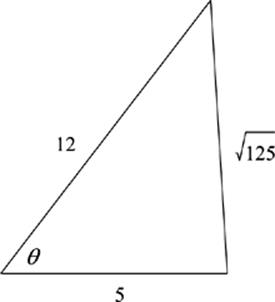
Figure 6
Note: Figure not drawn to scale.
39. In Figure 6, what is the value of θ in degrees?
(A) 62.00
(B) 65.38
(C) 65.91
(D) 68.49
(E) 68.70
40. If 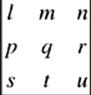 = A, then
= A, then  =
=
(A) 2A
(B) 4A
(C) 6A
(D) 8A
(E) 18A
41. In the function g(x) = A[sin (Bx + C)] + D, constants are represented by A, B, C, and D. If g(x) is to be altered in such a way that both its period and amplitude are increased, which of the following constants must be increased?
(A) A only
(B) B only
(C) C only
(D) A and B only
(E) C and D only
42. All of the elements of list M and list N are arranged in exactly 20 pairs, such that every element from list M is paired with a distinct element from list N. If in each such pair, the element from list M is larger than the element from list N, then which of the following statements must be true?
(A) The median of the elements in M is greater than the median of the elements in N.
(B) Any element of M is greater than any element of N.
(C) The mode of the elements in M is greater than the mode of the elements in N.
(D) The range of the elements in M is greater than the range of the elements in N.
(E) The standard deviation of the elements in M is greater than the standard deviation of the elements in N.
43. If 3, 5, 8.333, and 13.889 are the first four terms of a sequence, then which of the following could define that sequence?
(A) a 0 = 3; an + 1 = an + 2
(B) a 0 = 3; an + 1 = 2an − 1
(C) a 0 = 3; an = an + 1 + ![]()
(D) a 0 = 3; an = ![]() an − 1
an − 1
(E) a 0 = 3; an ![]() an − 1 −
an − 1 − ![]() an − 1
an − 1
44. If 0 ≤ n ≤ ![]() and cos(cos n) = 0.8, then tan n =
and cos(cos n) = 0.8, then tan n =
(A) 0.65
(B) 0.75
(C) 0.83
(D) 1.19
(E) 1.22
45. The height of a cylinder is equal to one-half of n, where n is equal to one-half of the cylinder"s diameter. What is the surface area of this cylinder in terms of n ?
(A) ![]()
(B) 2πn 2
(C) 3πn 2
(D) 2πn 2 + ![]()
(E) 2πn 2 + πn
46. If (tan θ − 1)2 = 4, then which of the following could be the value of θ in radian measure?
(A) −0.785
(B) 1.373
(C) 1.504
(D) 1.512
(E) 3
47. Which of the following expresses the range of values of y = g(x), if g(x) = ![]() ?
?
(A) {y: y ≠ 0}
(B) {y: y ≠ 1.25}
(C) {y: y ≠ −4.00}
(D) {y: y > 0}
(E) {y: y ≤ −1 or y ≥ 1}
48. If csc θ = ![]() , then where defined, cos θ =
, then where defined, cos θ =
(A) 3t
(B) ![]()
(C) ![]()
(D) 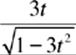
(E) 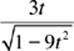
49. If f(x, y) = ![]() , then which of the following statements must be true?
, then which of the following statements must be true?
I. If x = 0 and y ≠ 0, then f(x, y) = 1.
II. If x = 1, then f(x, x) = 1.
III. f(x, y) = f(y, x)
(A) I only
(B) II only
(C) I and II only
(D) I and III only
(E) I, II, and III
50. A triangle is formed by the x-axis, the y-axis, and the line y = mx + b. If m = −b 3, then what is the volume of the cone generated by rotating this triangle around the x-axis?
(A) ![]()
(B) ![]()
(C) π
(D) 3π
(E) 9π
S T O P
IF YOU FINISH BEFORE TIME IS CALLED, YOU MAY CHECK YOUR WORK ON THIS TEST ONLY. DO NOT WORK ON ANY OTHER TEST IN THIS BOOK.
HOW TO SCORE THE PRINCETON REVIEW PRACTICE SAT MATH SUBJECT TEST
When you take the real exam, the proctors will collect your test booklet and bubble sheet and send your bubble sheet to New Jersey where a computer looks at the pattern of filled-in ovals on your bubble sheet and gives you a score. We couldn"t include even a small computer with this book, so we are providing this more primitive way of scoring your exam.
Determining Your Score
STEP 1 Using the answers on the next page, determine how many questions you got right and how many you got wrong on the test. Remember: Questions that you do not answer don"t count as either right answers or wrong answers.
STEP 2 List the number of right answers here.
STEP 3 List the number of wrong answers here. Now divide that number by 4. (Use a calculator if you"re feeling particularly lazy.)
STEP 4 Subtract the number of wrong answers divided by 4 from the number of correct answers. Round this score to the nearest whole number. This is your raw score.
STEP 5 To determine your real score, take the number from Step 4 above and look it up in the left column of the Score Conversion Table on this page; the corresponding score on the right is your score on the exam.
(A) ______________
(B) ______________ ÷ 4 = (C)______________
(A) ______________ − (C) ______________ = ______________
MATHEMATICS LEVEL 2 SUBJECT TEST FORM B
Answer Key
1. E
2. B
3. A
4. A
5. D
6. C
7. E
8. B
9. B
10. D
11. D
12. C
13. C
14. A
15. A
16. B
17. B
18. C
19. B
20. E
21. E
22. B
23. B
24. D
25. D
26. D
27. E
28. D
29. E
30. A
31. C
32. A
33. D
34. B
35. C
36. C
37. E
38. A
39. D
40. D
41. A
42. A
43. D
44. D
45. C
46. A
47. A
48. C
49. C
50. B
MATHEMATICS LEVEL 2 SUBJECT TEST SCORE CONVERSION TABLE

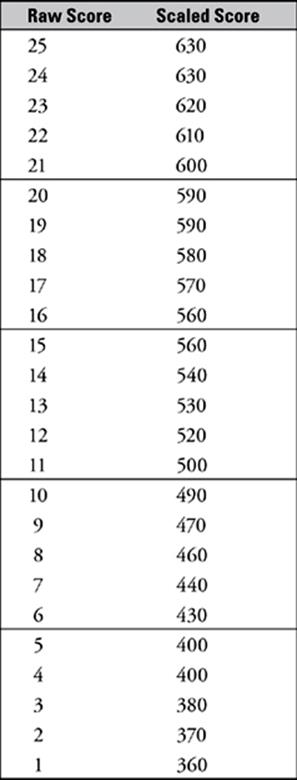

Mathematics Level 2 Test Form B Answers
Source: https://schoolbag.info/mathematics/sat_3/16.html
Posted by: sellarsvate1986.blogspot.com

0 Response to "Mathematics Level 2 Test Form B Answers"
Post a Comment